What is a pendulum?
A pendulum is a fascinating device that consists of a ball-shaped mass, known as the “bob,” suspended by a theoretically massless string from a fixed point. An extension of this is the coupled pendulum, which we shall study in a while. When you displace this bob from its starting position, it begins to swing back and forth in a smooth, predictable arc. This swinging, or oscillation, is driven by gravity, with each swing covering a distance called the “amplitude.” The time it takes for the pendulum to complete one full swing is known as the “time period.” The inverse of this value is called the “frequency of oscillation”, which indicates the number of oscillations of the pendulum per second. When set in motion, the pendulum swings continuously due to its inertia, maintaining a steady rhythm that has proven invaluable in timekeeping.
How do pendulums work?
Interestingly, a pendulum’s time period and frequency depend on the length of the string and the pull of gravity. They do not depend on the bob’s mass. For example, if you replace a sponge ball with a metal gong, their time period will remain the same. This happens regardless of their difference in mass, as long as the length of the string and the acceleration due to gravity remain the same.
The formula for time period T (and frequency f) shows why this is the case.
T= 1/f=2π√L/g
Where L=length of the string and g= acceleration due to gravity. Hence these are the factors that affect T and f.
What is a coupled pendulum?
In a coupled pendulum setup, two simple pendulums are connected by a string or a solid rod, creating a fascinating interaction. This connection introduces complexity, making it ideal for studying unique physical phenomena. A single pendulum traditionally keeps time in classic clocks. However, a pendulum setup can also measure gravitational acceleration. This acceleration varies slightly across different locations on Earth. By observing variables like the period (T) and the length (L) of the pendulum, we can calculate gravitational acceleration precisely using the known formula given above. However, a coupled pendulum can be an excellent tool for studying and verifying various physical phenomena, as we’ll explore below.
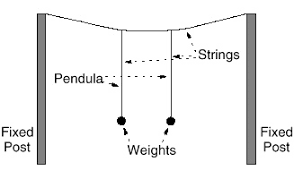
A coupled pendulum. Source- Physics Forums
Theory of a coupled pendulum
It beautifully demonstrates several physical phenomena. When you set one pendulum in motion by displacing it from its resting position, it continues to oscillate due to inertia of motion. This motion then influences the second pendulum, causing it to oscillate as well. Interestingly, as the first pendulum’s amplitude gradually decreases, the second pendulum’s amplitude increases. This transfer continues until the second pendulum oscillates with the same amplitude as the first. However, the process reverses, leading to a back-and-forth exchange of energy between the two pendulums. Eventually, this alternating motion fades, and both pendulums come to a stop.
Verifying theories of physics with coupled oscillation
This helps us demonstrate the following physical phenomenon-
Resonance in a coupled pendulum
When you set a pendulum in motion, it oscillates at a specific, unchanging frequency, known as its resonant frequency. Interestingly, even if you increase the amplitude (the height of each swing), the time period or frequency remains constant. This frequency is determined solely by the length of the string. For any pendulum with a fixed length, the oscillation frequency stays the same, regardless of amplitude.
The resonant frequency is a natural frequency that’s unique to a particular object—like this pendulum. When an object oscillates at its resonant frequency, it reaches maximum oscillation. At resonance, energy transfers most efficiently between objects vibrating at the same frequency, creating the strongest and most sustained oscillations.
A coupled pendulum setup demonstrates this concept clearly. Since both pendulums have the same string length, they oscillate at the same resonant frequency, allowing them to resonate together effectively. When the first pendulum begins to oscillate, it transfers energy through the connecting beam at the top. This energy transfer is highly efficient because it occurs at their resonant frequency.
As the first pendulum swings, it causes the second pendulum to increase in amplitude. This process continues until all the energy from the first pendulum is transferred to the second. At that point, the second pendulum oscillates with the same amplitude as the first. This complete transfer of energy showcases the power of resonance, which the fixed lengths of both pendulums in this setup make possible.
Conservation of energy in a coupled pendulum
The law of conservation of energy states that energy can transform from one form to another, but it cannot be created or destroyed. The coupled pendulum clearly demonstrates this principle. As the first pendulum transfers energy to the second, and vice versa, we observe the amplitudes of both pendulums gradually decrease over time. Energy transfers efficiently during this exchange. However, it’s important to note that there is a loss of energy in the process.
Friction with the air causes some energy to dissipate as heat, leading to a loss of energy rather than a full transfer between the pendulums. The total energy within the system remains conserved, meaning it doesn’t vanish. However, it transforms into other forms, such as thermal energy due to friction. Therefore, while the law of conservation of energy holds true, friction and heat generation affect the dynamic system. They contribute to the overall energy transformations at play.
Potential energy to kinetic energy in a swinging pendulum
When you raise the pendulum to a certain height and displace it to the side, it gains potential energy (PE). As the pendulum oscillates and reaches the lowest point in its swing, the system completely converts potential energy into kinetic energy (KE). At the highest points of oscillation, potential energy is highest, and the pendulum is momentarily at rest. Here, the kinetic energy is lowest. At the lowest point of its swing, kinetic energy is at its maximum, while potential energy is at its minimum. This continuous transformation between potential and kinetic energy is a fundamental aspect of the pendulum’s motion. It illustrates the principles of energy conservation in action.
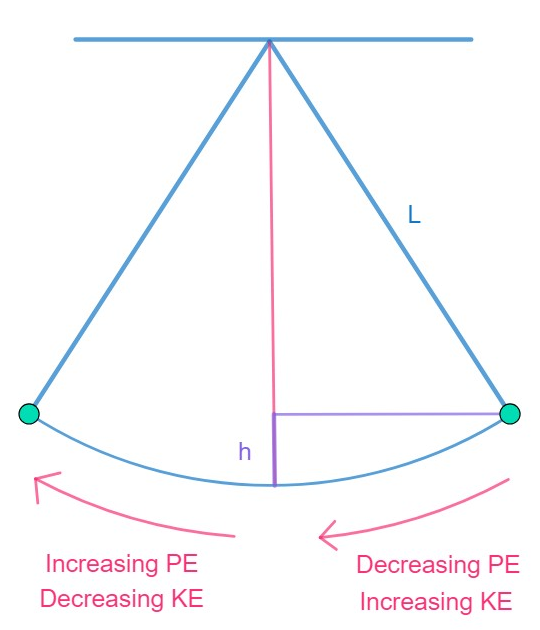
Transformation of potential energy into kinetic energy and vice versa in a pendulum. Source – StudySmarter
Wave motion of a swinging pendulum
Wave motion involves the repeated oscillation of particles. Sound waves arise from the oscillation of air particles. Water waves, such as transverse waves, form when we drop a pebble into water. The pendulum also exhibits wave motion, and this concept becomes evident when we explore it further. Our blog on wave motion explains this in detail. The image provided here illustrates the oscillations of a pendulum about its mean position.
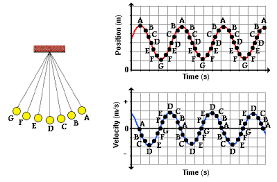
The wave motion of a pendulum. Source- The Physics classroom.
Why do you need a coupled pendulum?
The coupled pendulum demonstrates the laws of physics beautifully, showcasing multiple phenomena. These concepts are often abstract and challenging to visualize. However, this pattern and blog aim to make them clearer for students. To support this, Labkafe has created a coupled pendulum model that allows you to demonstrate four unique, interdisciplinary concepts in physics. These demonstrations help students strengthen their ability to make connections across various principles.
Contact our lab experts today to get amazing demonstration models for your science classrooms!







Leave a Reply